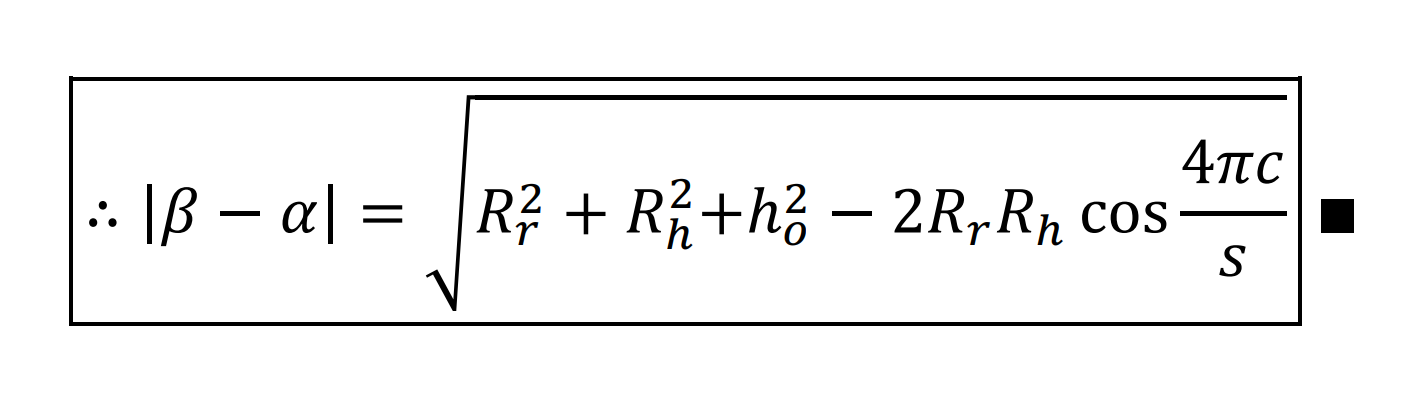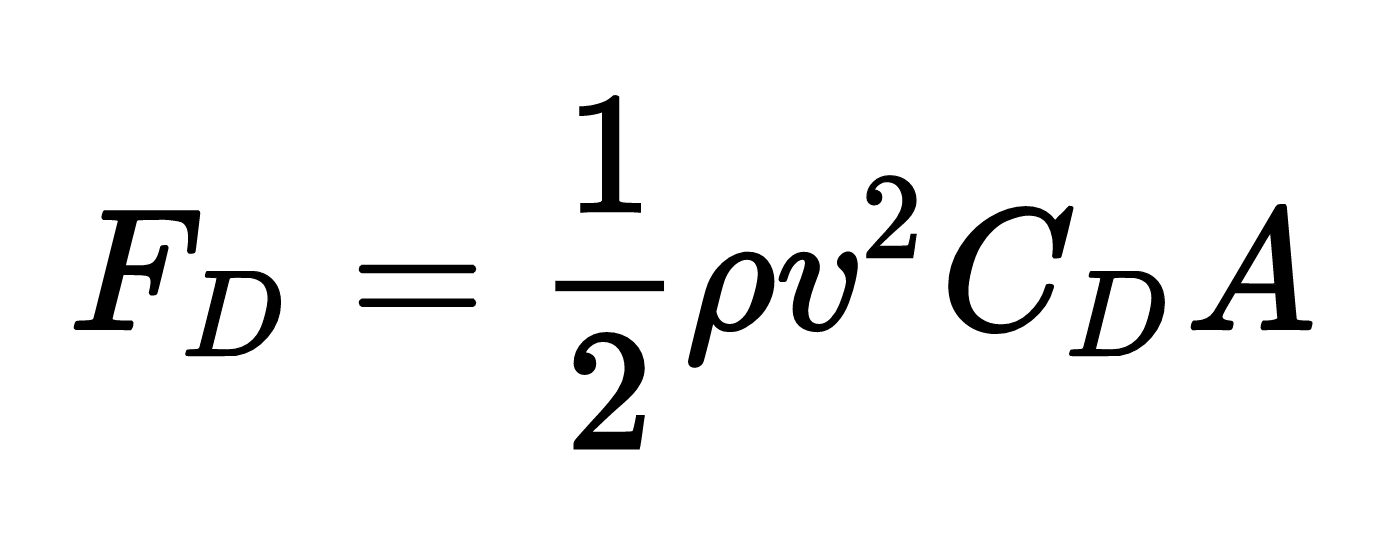Should I seriously consider radial lacing?

Question

Summary: Radial lacing looks cool, but is it better?

Not expecting bicycles.SE to be the right forum, I asked about the dynamics of spokes on Engineering.SE and didn't get a satisfying answer. I then confirmed on Physics.SE that my reasoning is accurate. I'm curious now not about the theory but about the applications, or the implications of a nonrigid wheel. Rigidity is relative. No wheel is perfectly rigid. Once loaded any bicycle wheel becomes an ellipse.

Spokes can be laced in two fashions:

1. Tangent lacing build an almost-rigid wheel. The additional tension in the spokes is immediately activated when a torque is applied at the hub, from either braking or the drivetrain.
2. Radial lacing, which is increasingly seen on the market, requires the hub to first rotate a minuscule amount before the additional forces in the spokes are activated. Those additional forces transmit the torque to the rim.

Sheldon Brown points out:

This ease of turning increases the risk of [spokes] unscrewing themselves on the road.

as an advantage to tangent lacing. I speculate another factor. When replacing tangent lacing with radial lacing, we are trading one weakness (the rub at each J-bend will sooner or later wear out a spoke) with another (the activation torque needed in radial lacing moves each spoke on both ends at each torque application, eventually also wearing the joint).

Oddly, Sheldon Brown states:

He held the front brake and pushed down on a pedal with his foot. The spokes of the rear wheel changed angle noticeably, pinging as they rotated in the spoke holes of the hub, and ringing with rising musical pitch like an electric guitar when the player pulls up on the tremolo bar.

and yet we do have precisely that: radial lacing on front wheels with disc brakes, which causes an even greater torque than the drivetrain, and higher even with hydraulic brakes.

Preliminaries

1. The tension in radial spokes ought to be considerably higher than on tangent spokes in a traditional arrangement. The spokes on radially spoked wheels seem considerably thicker, and this suggests that an increased tension is expected. A much higher tension also means that the "activation" angle is far smaller, leading to a more solid wheel. But the confirmation of this reasoning by some cyclists who rode radially laced wheels is debunked by Jobst Brandt.
2. Likewise, given the "activation rotation", we would expect a radially laced wheel to be "mushy" under torque. This would be an advantage, acting like a shock absorber. Again debunked (same reference).

Questions (two simple questions):

3. The hubs in radial lacing are more substantial than the hubs on tangentially spoked wheels, as they should. What are the weight (and longevity?) implications?
4. I understand that whether a wheel has cup-and-cone or sealed bearings in its hub is orthogonal to whether it is laced in the old or the new style. Can you confirm this is true?

Please write 3/4 in your answer. Feel free to comment on 1/2.

Bonus fifth (feel free to ignore this part):

5. I want to buy a wheelset and ride it for thousands of kilometers with very routine, mundane maintenance with off-the-shelf components in my (modest) home shop. Which of the two types of lacings is the better bet?

When I understand enough, I'll be able to answer this (fifth) for myself, as well as the following sixth question: which is easier to build at home, a radially spoked or a tangentially spoked wheel?

Notes:

1. Even under radial lacing, the rear wheel continues to be laced in the traditional method on the drive side. Only the nondrive side takes the newer lacing style.
2. We ignore in this question rim brakes.
3. We ignore in this question the asymmetrical forces (right side vs left side) on the spokes from turning on flat terrain, if any.

Related:

• Are small radially laced motor hub wheels like Swytch trash?
• Any experience about radially-laced hub flanges actually breaking?
• Will disc brakes aggravate the problem with spokes pulling through the rim?
• Trying to understand the impact of different tensions between inner and outer spokes
• How is the finishing stage of truing a laced wheel improved by adjusting spokes in groups of 4?
• Wheelbuilding at Sheldon Brown

References:

I have just become aware of the following references:

• The Art of Wheelbuilding
• The Bicycle Wheel
• Sutherland's Handbook for Bicycle Mechanics, 1; 2

Answer 1

I just happened to have recently written a little paper on the spoke length formula, so with my knowledge about wheels refreshed, I think I'll take a shot at answering this question. Let me approach it in parts.

---

Radial Versus Tangential

As noted by other answers already, the primary advantages are a slightly shortened spoke length and ease of wheelbuilding. I think the numerous disadvantages far outweigh these factors though. You have a pretty good understanding of how tangentially-spoked wheels respond to various inputs, so I won't elaborate there unless you want me to.

By how much are the spokes shortened, one might ask? Not that much at all. For reference, here's the spoke length formula (my work):

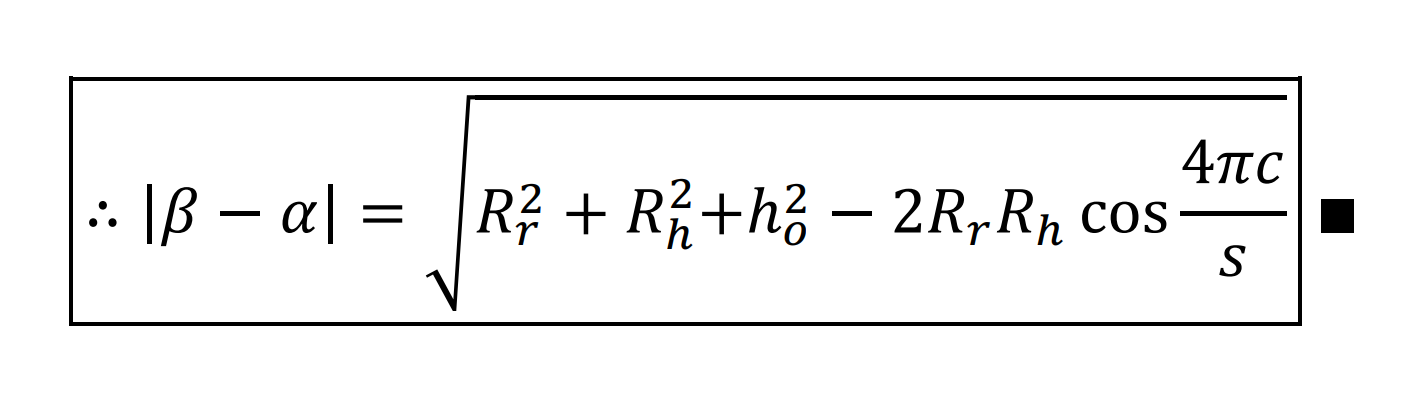
As you can see, the crossing number c is only present inside the argument of the cosine function. The term attached to the cosine function is fairly small because its coefficient (twice the product of the rim radius and hub radius) is small by itself compared to the square of the rim radius. By substituting the arbitrary values of rim radius R_r = 300mm, hub radius R_h = 30mm, hub flange offset h_o = 50mm, and spoke count s = 32, we get a length of 294.1mm for a 3-cross wheel, and 274.6mm for a radially-spoked one. The difference in length is 19.5mm, which translates to a mass difference of approximately 15.5g for 2mm round stainless steel spokes. Nice.

And as for air drag? Here's the idealistic drag equation (screenshotted from Google):

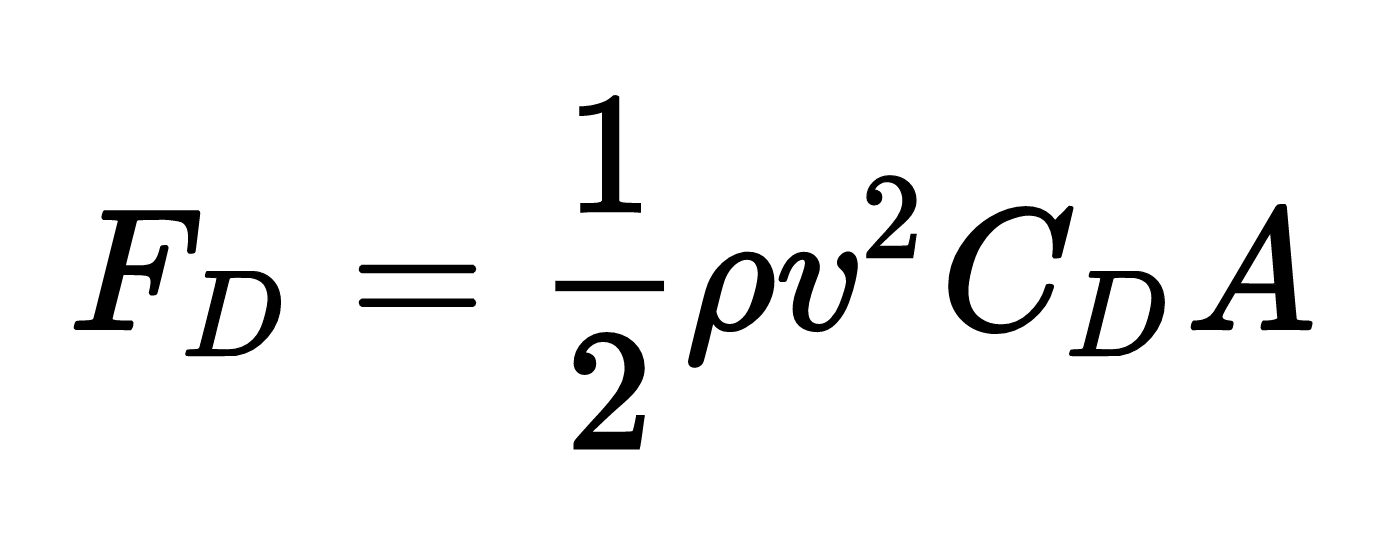
What we are concerned about here is A, the cross-sectional area. Everything else is a constant if we are comparing two otherwise identical spokes of different lengths. Using the simplification of spokes intersecting the airstream perpendicularly (so velocity is constant), I calculate a reduction of cross-sectional area of 6.5% from an already insignificant value. Nice.

Evidently, this point is fairly moot.

I don't think the time savings is particularly important either. It doesn't take that long to spoke either style of wheel; the primary time expenditure is on tensioning and trueing.

Your other two questions on the other SE websites.

On the Engineering SE, you asked:

How can wheels with spokes concurrent at the center be solid?

I think you know the answer to this question already: no real-life item is perfectly stiff. Since you correctly mention that spokes have negligible lateral stiffness, a radially-built bicycle wheel is indeed going to suffer when considering twists of the hub relative to the rim. We see that a tangent line to the hub flange drawn where any spoke meets it is in fact perpendicular to the spoke, so the spoke is unable to handle lateral loads. In order for a spoke to provide/resist a torque at the hub, it must deflect to change the spoke-hub angle. Unfortunately, for this to happen, the spoke must lengthen to a great extent as the hypotenuse grows, enough to break it if non-trivial torque is applied.

You then note,

Of course a bicycle wheel is so light precisely because it is not a solid object, but the rim and hub have no relative "wobble".

I think this is a slightly skewed way of looking at it. The reason a wire-spoked wheel is so lightweight for its load capacity is that material is only present where its needed, and this material is being used only in its "best", most efficient directions. The rim and hub are relatively stable because there are many forces of large magnitude (spoke tension) acting on the pair which hold them in their most stable position relative to one another.

Virtually the same question is asked on the Physics SE website.

---

Let me now address your five main points:

1. Tension

The tension in radial spokes ought to be considerably higher than on tangent spokes in a traditional arrangement. The spokes on radially spoked wheels seem considerably thicker, and this suggests that an increased tension is expected. A much higher tension also means that the "activation" angle is far smaller, leading to a more solid wheel.

I must begin by noting I have no first-hand experience with radial wheels. That said, I don't think there will be any significant tension difference; for starters, I don't tension my 2× versus 3× wheels any differently. This is confirmed by Park Tool's conversion table for use with their tension meter: there is no separate category for radial lacing.

I think a lot of radially-spoked wheels use aerodynamic bladed spokes, so they may appear thicker when viewed from the side. Furthermore, note that spoke thickness is largely independent of expected tension. It is common practice to use purposefully thinner spokes (eg. Juhist's beloved DT Swiss Alpine III) to make the spokes stretch more for the same tension, giving a more evenly tensioned wheel.

2. Stiffness

Likewise, given the "activation rotation", we would expect a radially laced wheel to be "mushy" under torque. This would be an advantage, acting like a shock absorber.

I think any significant mushiness would be immediately followed by catastrophic wheel failure as the spokes elongate past their breaking point. When you accelerate the bike, the wheels are expected to spin [citation needed]. Hence, even if there is, say, 10° of mushiness, the wheel is going to be rotating several full turns (several thousand degrees of rotation) very shortly.

Even if this effect were real and significant, it would certainly not act like a shock absorber, at least not in a useful way. Remember the key to an effective shock absorber is the combination of a spring and damper. You only have a spring here. Considering how most people except for Jan Heine are chasing stiffer frames, having an undamped spring in your acceleration parts train is not beneficial.

3. Hubs

The hubs in radial lacing are more substantial than the hubs on tangentially spoked wheels, as they should. What are the weight (and longevity?) implications?

I don't believe J-bend hubs are sold as radial-specific, so that negates some aspects of this topic already. As @Michael points out in the comments though, some straight-pull hubs are radial-specific by nature of their design, so you must be careful about that. Hubs in radially-spoked wheels are almost certainly at a disadvantage for strength and long-life though. In a tangentially-spoked wheel, the spokes tend to pull against opposing ones in a way that almost cancels out the net tension felt by the hub flanges. Contrarily, with a radially-spoked wheel, every spoke is attempting to pull the hub flange off the hub, so to speak. Sheldon's article you link to mentions this aspect. In their product manuals, Shimano and other hub manufacturers explicitly forbid radially lacing their J-bend hubs, further supporting this argument.

4. Bearings

I understand that whether a wheel has cup-and-cone or sealed bearings in its hub is orthogonal to whether it is laced in the old or the new style. Can you confirm this is true?

Yeah, bearing type is independent on lacing style because again, the common J-bend hub is not sold as radial-specific or tangential-specific (bar some straight-pull models as mentioned previously). I'd also like to highlight the terminology "old or new style"; I'm pretty sure both styles of lacing have been prevalent throughout history, so neither is notably newer or older.

5. Bonuses

I want to buy a wheelset and ride it for thousands of kilometers with very routine, mundane maintenance with off-the-shelf components in my (modest) home shop. Which of the two types of lacings is the better bet?

I think tangential lacing gives better service life, if not for the spokes then at least for the hub. Hence, that's the option I would recommend. The time savings of radial lacing is not worth it. For your second bonus question, I'd say radial is easier because you don't have to keep track of crossings, nor the location of the key spoke.

---

More bonuses!

I speculate another factor. When replacing tangent lacing with radial lacing, we are trading one weakness (the rub at each J-bend will sooner or later wear out a spoke) with another (the activation torque needed in radial lacing moves each spoke on both ends at each torque application, eventually also wearing the joint).

I don't think so. Rubbing at the J-bend occurs for both tangential and radial lacing patterns. Either way, I think it will be the soft aluminum hub which loses the battle, not the steel spoke.

In reference to Sheldon's anecdote (well, technically John's) about a radially laced rear wheel, you write:

[A]nd yet we do have precisely that: radial lacing on front wheels with disc brakes...

Radial lacing is only ever used with rim brakes. In the context of disc-braked wheels, [radial] and [successful]: pick one.

A brake's ultimate goal is to provide a force at the point of wheel-ground intersection that is parallel to the ground and inline with your direction of movement. Disc brakes achieve this by applying a torque to the hub, which transmits it to the rim via the spokes, and then the rim applies a force to the ground. With rim brakes, the rim effectively pivots around the brake in an arc towards the rear of the bike, directly generating this braking force. The hub and spokes do not feel any torque, but rather only a linear force towards the back of the bike which slows the rider.

---

_{As a side note, when discussing such scientific matters like this in our native English, let's be precise in our use of language. Spokes are not "concurrent" to the hub, they are radial. I also think the notion of "inserting" tension into a spoke is a little absurd.}

Answer 2

I think your detailed question shows one flaw, a lack of understanding why radial spoking is used and what circumstances tangental spoking must be used.

Radial lacing is used almost exclusively with rim brakes on front wheels. It provides a tiny aerodynamic advantage and a tiny weight saving against 2x or 3x patterns because the spokes are shorter. The spokes can be any type, even Sapim Laser, but the spoke nipples need to have some retaining compound on the threads as there is a higher chance of these coming unwound when they are out of tension (wheel load, impact) without.

Radial lacing is also seen used for one side of either the rear wheel and one side of very few disc brake front wheels, where it serves to look cool, generally on a high end race wheel where marginal gains and psychological gains can be important.

If you lace a rear hub, a hub motor or a braked hub (disc, drum etc) with a radial pattern on both sides, the spokes will quickly lose tension as the load changes angle on them all the time. There a small wheel e-bike conversions that suffer exactly this problem. Even a 1x pattern is sufficient to brace against most of the load, allowing for a more robust wheel, but 2x or 3x is preferred.

Radial has one distinct advantage, it's very quick to lace a wheel. However, it's no quicker to tension and true so it's only a small saving.

For the application you describe, you absolutely cannot use radial spoking.

Answer 3

Radial lacing should be avoided for two reasons.

Firstly, to get a durable wheel, you need massive tension in all spokes. In radially laced wheels, all of this tension acts to destroy the hub flange. There's a very large probability that the hub flange will fail, unless you tensioned the spokes so little that the wheel fails due to a total and complete loss of tension in all spokes. In tangentially spoked wheels, the tensions cancel each other at the hub flange. However, we don't use perfectly tangentially tensioned wheels, only approximately so (36 spokes, cross 3) but that's enough to keep the destruction potential at the hub flange at a minimum. Only a part of the tensions in cross-3 36-spoke wheels is non-cancelling.

Secondly, in radial lacing you don't interlace the crossing spokes. In non-radial wheels, you do. This is important because the bottommost spoke starts to lose some tension in a radially laced wheel. In tangentially laced wheels, this loss of tension means the another spoke in that interlaced pair loses tension too, which distributes the tension loss in a better way across a larger number of spokes. Thus, at the same level of tension, a crossing spoke pattern (assuming interlaced spokes) is more durable: it can carry heavier load before the spoke nipples start to self-unscrew.

So you lose big time. If you could ever use the same tension in radially laced wheels than you use in cross-3 wheels, your wheel would be still less durable. But you can't even use the same tension in radially laced wheels!

I'm sure plenty of racing cyclists will heavily downvote me. Let me point out that such racing cyclists probably weigh 50 kg, ride a bike that weighs 7 kg, and never attach panniers to their bike.

Before someone claims that front wheels carry less load than rear wheels, and that radial spoking is used only for the less-loaded front wheel so "it should be fine", let me disagree: front wheel has to be capable of carrying 100% of the weight of the bike, the rider and the cargo. That happens when braking at the hardest possible rate, so that the rear wheel loses all its load.

Answer 4

No +

Radial is okay on a Ford Model T car wheel, or a horse-pulled wagon or on a very small wheel from a children's bike.

Not good for a normal bike with any kind of brake.